|
15 |
PMHF導出法の変更 (4) |
LAT1⇒DPF2の平均PUDの計算
次にLAT1からDPF2の平均PUDを計算します。同様に、LAT1の状態確率が前稿#105と比べて変化します。具体的にはIFのVSG preventable部分の確率が下がります。
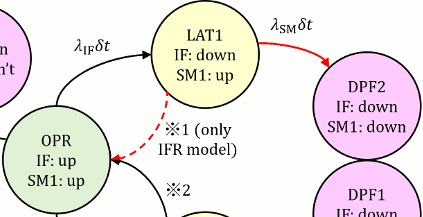
前稿#105の式(105.1)はそのままです。LAT1からDPF2への平均PUDは、 $$ \begin{eqnarray} \overline{q_{\mathrm{DPF2,IFR}}}&=&\frac{1}{T_\text{lifetime}}\Pr\{\mathrm{DPF2\ at\ }T_\text{lifetime}\}\\ &=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\Pr\{\mathrm{LAT1\ at\ }t\cap\mathrm{SM\ down\ in\ }(t, t+dt)\}\\ &=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\Pr\{\mathrm{SM\ down\ in\ }(t, t+dt]\ |\ \mathrm{LAT1\ at\ }t\}\\ & &\ \ \ \ \cdot\Pr\{\mathrm{LAT1\ at\ }t\} \end{eqnarray} \tag{363.1} $$ LAT1の状態確率に対する条件を求めます。IFのフォールトのうちMPF detectedはlatentとならず、直ちに修理されるものとみなされるため、LAT1は
- IFの不稼働状態、かつ
- SM1によりVSGは抑止され、かつSM1により検出されず、かつ
- SM2により検出されず、かつ
- SM1の稼働状態
のようにこの条件が追加されます。これを確率式で書くと以下のように赤字の条件が加わります。さらに(355.1)を用いて書き換えると、 $$ \Pr\{\mathrm{LAT1\ at\ }t\}=\Pr\{\mathrm{IF\ down\ at\ }t\cap\text{IF preventable}\\ \cap\color{red}{\text{IF not detected }}\cap\mathrm{SM\ up\ at\ }t\}\\ =\Pr\{\mathrm{IF^R\ down\ at\ }t\}\Pr\{\text{IF preventable}\}\color{red}{\Pr\{\text{IF not detected}\}}\Pr\{\mathrm{SM\ up\ at\ }t\}\\ =K_{\mathrm{IF,RF}}\color{red}{(1-K_\text{IF,det})}Q_{\mathrm{IF}}(t)A_{\mathrm{SM}}(t)\tag{363.2} $$ と書けます。
一方、 $$ \require{cancel} \Pr\{\mathrm{SM\ down\ in\ }(t, t+dt]\ |\ \mathrm{LAT1\ at\ }t\}\\ =\Pr\{\mathrm{SM\ down\ in\ }(t, t+dt]\ |\\ \mathrm{SM\ up\ at\ }t\cap\bcancel{\mathrm{IF^R\ down\ at\ }t}\cap\bcancel{\text{IF preventable}}\cap\bcancel{\color{red}{\text{IF not detected}}}\}\\ =\Pr\{\mathrm{SM\ down\ in\ }(t, t+dt]\ |\ \mathrm{SM\ up\ at\ }t\}=\lambda_{\mathrm{SM}}dt\tag{363.3} $$ であるから、(363.1)は、 $$ \begin{eqnarray} \overline{q_{\mathrm{DPF2, IFR}}}&=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}K_{\mathrm{IF,RF}}\color{red}{(1-K_\text{IF,det})}Q_{\mathrm{IF}}(t)A_{\mathrm{SM}}(t)\lambda_{\mathrm{SM}}dt\\ &=&\frac{K_{\mathrm{IF,RF}}\color{red}{(1-K_\text{IF,det})}}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\left[(1-K_{\mathrm{IF,MPF}})F_{\mathrm{IF}}(t)+K_{\mathrm{IF,MPF}}F_{\mathrm{IF}}(u)\right]\\ & &\cdot\left[(1-K_\text{SM,MPF})f_\text{SM}(t)+K_\text{SM,MPF}f_\text{SM}(u)\right]dt\\ &\approx&\frac{K_{\mathrm{IF,RF}}\color{red}{(1-K_\text{IF,det})}}{2}\lambda_{\mathrm{SM}}\lambda_{\mathrm{IF}}\left[(1-K_{\mathrm{MPF}})T_\text{lifetime}+K_{\mathrm{MPF}}\tau\right]\\ &=&K_{\mathrm{IF,RF}}\color{red}{(1-K_\text{IF,det})}\beta, \end{eqnarray}\tag{363.4} $$
$$ ただし、\begin{cases} \begin{eqnarray} u&:=&t\bmod\tau\\ \beta&:=&\frac{1}{2}\lambda_{\mathrm{IF}}\lambda_{\mathrm{SM}}[(1-K_{\mathrm{MPF}})T_\text{lifetime}+K_{\mathrm{MPF}}\tau]\\ K_{\mathrm{MPF}}&:=&K_{\mathrm{IF,MPF}}+K_{\mathrm{SM,MPF}}-K_{\mathrm{IF,MPF}}K_{\mathrm{SM,MPF}}\\ \end{eqnarray}\end{cases} $$
RAMS 2022においてMPF detectedの再考に基づくPMHF式の論文発表が終了したため、秘匿部分を開示します。
