前稿#355の続きです。変更方針はMPF latentに分類していたMPF detectedを即時修理とするものです。従って、MPF detectedは故障しないことと等価です。

OPR⇒SPFの平均PUDの計算

従来はMPF detectedをMPF latent扱いにしていたものを、MPF detectedに変更しました。MPFの意味はVSG preventableなIFのフォールトであるため、SPFの計算に影響はありません。従って、以下は前稿#103と同様です。

OPRステートからSPFステートへの平均PUD(66.13)を計算します。

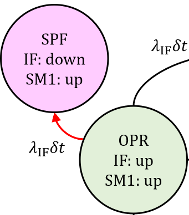
OPRからSPFへの平均PUDは、 $$ \begin{eqnarray} \overline{q_{\mathrm{SPF,IFU}}}&=&\frac{1}{T_\text{lifetime}}\Pr\{\mathrm{SPF\ at\ }T_\text{lifetime}\}\\ &=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\Pr\{\mathrm{OPR\ at\ }t\cap\mathrm{IF\ down\ in\ }(t, t+dt]\cap\overline{\mathrm{IF\ preventable}}\}\\ &=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\Pr\{\mathrm{IF\ down\ in\ }(t, t+dt]\ |\ \mathrm{OPR\ at\ }t\}\\ & &\ \ \ \ \cdot\Pr\{\mathrm{OPR\ at\ }t\}\Pr\{\overline{\mathrm{IF\ preventable}}\} \end{eqnarray} \tag{361.1} $$ ここでOPRは、 $$ \begin{eqnarray} \Pr\{\mathrm{OPR\ at\ }t\}&=&\Pr\{\mathrm{IF\ up\ at\ }t\cap\mathrm{SM\ up\ at\ }t\}=\Pr\{\mathrm{IF^U\ up\ at\ }t\}\Pr\{\mathrm{SM\ up\ at\ }t\}\\ &=&R_\mathrm{IF}(t)A_\mathrm{SM}(t)\end{eqnarray}\tag{361.2} $$

一方、(361.1)の右辺積分中の条件付き確率式は、 $$ \Pr\{\mathrm{IF^U\ down\ in\ }(t, t+dt]\ |\ \mathrm{OPR\ at\ }t\}\\ =\Pr\{\mathrm{IF^U\ down\ in\ }(t, t+dt]\ |\ \mathrm{IF^U\ up\ at\ }t\cap\text{SM up at }t\}\\ =\Pr\{\mathrm{IF^U\ down\ in\ }(t, t+dt]\ |\ \mathrm{IF^U\ up\ at\ }t\}=\lambda_{\mathrm{IF}}dt \tag{361.3} $$ よって平均PUDは、 $$ \begin{eqnarray} \overline{q_{\mathrm{SPF,IFU}}}&=&\frac{1}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}(1-K_{\mathrm{IF,RF}})R_\mathrm{IF}(t)A_\mathrm{SM}(t)\lambda_{\mathrm{IF}}dt\\ &=&\frac{1-K_{\mathrm{IF,RF}}}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\left[1-Q_\text{SM}(t)\right]f_{\mathrm{IF}}(t)dt\\ &=&\frac{1-K_{\mathrm{IF,RF}}}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}f_{\mathrm{IF}}(t)dt-\frac{1-K_{\mathrm{IF,RF}}}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}Q_\text{SM}(t)f_{\mathrm{IF}}(t)dt\\ &=&\frac{1-K_{\mathrm{IF,RF}}}{T_\text{lifetime}}F_\text{IF}(T_\text{lifetime})\\ & &-\frac{1-K_{\mathrm{IF,RF}}}{T_\text{lifetime}}\int_0^{T_\text{lifetime}}\left[(1-K_\text{SM,MPF})F_\text{SM}(t)+K_\text{SM,MPF}F_\text{SM}(u)\right]f_{\mathrm{IF}}(t)dt,\\ & &\text{ただし、}u:=t\bmod\tau \end{eqnarray} \tag{361.4} $$ よって、$F_\text{SM}(t)=1-e^{-\lambda_{\mathrm{SM}}t}\approx\lambda_{\mathrm{SM}}t$と近似し、 $$ \begin{eqnarray} (361.4)&\approx&(1-K_{\mathrm{IF,RF}})\lambda_{\mathrm{IF}}-\frac{1-K_{\mathrm{IF,RF}}}{2}\lambda_{\mathrm{IF}}\lambda_{\mathrm{SM}}[(1-K_{\mathrm{SM,MPF}})T_\text{lifetime}+K_{\mathrm{SM,MPF}}\tau]\\ &=&(1-K_{\mathrm{IF,RF}})\lambda_{\mathrm{IF}}-(1-K_{\mathrm{IF,RF}})\alpha,\\ & &\text{ただし、} \alpha:=\frac{1}{2}\lambda_{\mathrm{IF}}\lambda_{\mathrm{SM}}[(1-K_{\mathrm{SM,MPF}})T_\text{lifetime}+K_{\mathrm{SM,MPF}}\tau] \end{eqnarray} \tag{361.5} $$

RAMS 2022においてMPF detectedの再考に基づくPMHF式の論文発表が終了したため、秘匿部分を開示します。

前のブログ 次のブログ