「ISO 26262第2版解説書」(日本規格協会)のPMHF式の続きです。
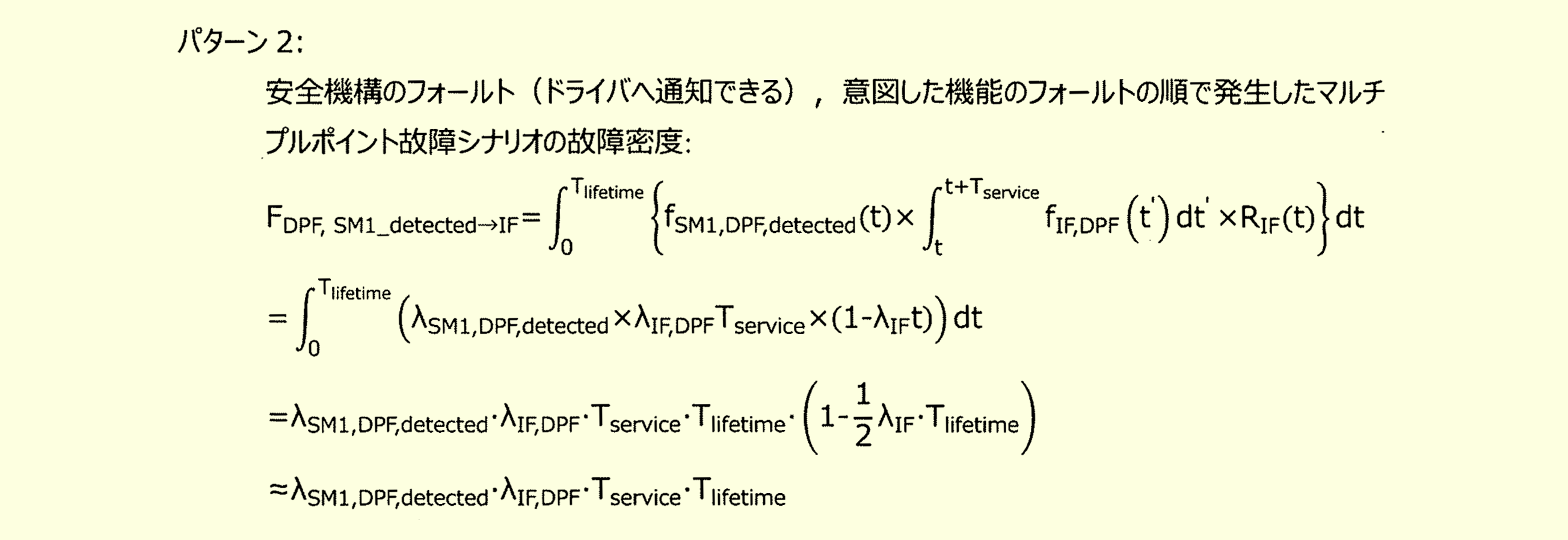
パターン3, 4
IFとSMを入れ替えることで、同様にパターン3, 4を導き、パターン1から4までを加えれば、
$$
M_\text{PMHF}\approx\frac{1}{2}\lambda_\text{IF,DPF}\lambda_\text{SM,DPF,lat}T_\text{lifetime}\\
+\frac{1}{2}\lambda_\text{IF,DPF}\lambda_\text{SM,DPF,det}T_\text{service}\\
+\frac{1}{2}\lambda_\text{SM,DPF}\lambda_\text{IF,DPF,lat}T_\text{lifetime}\\
+\frac{1}{2}\lambda_\text{SM,DPF}\lambda_\text{IF,DPF,det}T_\text{service}
\tag{313.1}
$$
となりそうです。
規格の問題点
ところが、規格は最初のパターン分けから間違っているので、これは誤った式です。
なぜなら、IFがフォールトして、検出・修理される、次にSMがフォールトして、検出・修理されるというパターンやその逆パターンが、この4パターンには含まれていないためです。規格に従えば、一度フォールトしたエレメントは検出・修理を繰り返し、レイテント状態において相手のエレメントがフォールトする場合にのみDPFとして扱います。
規格のパターン分けは、パターン1,2とパターン3,4の組にはっきりと分かれており、
- パターン1, 2: {SMがフォールトし検出・修理}が1以上の任意回、{IFのフォールト}
- パターン3, 4: {IFがフォールトし、検出・修理}が1以上の任意回、{SMのフォールト}
という特殊例しか数え上げていないのです。リペアラビリティで表せば、
- パターン1, 2: SMがリペアラブル、IFがアンリペアラブル⇒IFUモデル
- パターン3, 4: IFがリペアラブル、SMがアンリペアラブル⇒SMUモデル
という状態を表しています。
 図313.1 規格CTMC図
図313.1 規格CTMC図
これをCTMCで示したものが図313.1です。パターン1,2ではIFがアンリペアラブル固定(検出・修理はされない)、パターン3,4ではSMがアンリペアラブル固定(検出・修理はされない)という、余分な制約がかかっています。
本来は、初期状態でIFとSMは共にリペアラブルであり、かつ相手がフォールト時には自分がアンリペアラブルとなるという、マルコフ図のような動作となります。
結果として規格に依れば、パターン1, 2ではIFのリペア、パターン3, 4ではSMのリペアの考慮が抜けているため、PMHFを過大評価することになります。故障確率の過大評価は過小評価よりは良いかもしれませんが、逆にEOTTIが厳しくなるという弊害が生じます。
なお、本稿はRAMS 2024に投稿予定のため一部を秘匿していますが、論文公開後の2024年2月頃に開示予定です。
 前のブログ
次のブログ
前のブログ
次のブログ