|
26 |
論文読者からのご質問 |
台湾の読者からご質問を頂きました。原文は英語です。
論文"Generic Equations for a Probabilistic Metric for Random Hardware Failures According to ISO 26262"において、以下の2か所の式変形過程が分からないが、どうして次の式(13), (15)が導出されるのか?
(15) 省略、次ページで解説
当該論文の発表記事は#199です。そして式(13)の導出はブログに書かれていない部分の式変形です。そのため、今回新たにChatGPTに導出させたのでそれを示します。メールに英語で回答する都合上ChatGPTと英語で会話したので、併せて和訳を付けます。ChatGPTには元の式(13)の一部 $$ F_\text{SM}(T_\text{service})\sum_\text{i=0}^{n-1}R_\text{SM}(t-(i+1)T_\text{service}) $$ について変形させました。
ChatGPT:
The original equation is:
元の方程式は以下のとおりである。:
$$
(1-R_\text{SM}(T_\text{service}))\sum_\text{i=0}^{n-1}R_\text{SM}(t-(i+1)T_\text{service})
$$
First, we apply the distributive law. This involves multiplying $1-R_\text{SM}(T_\text{service})$ with each term inside the summation. Doing so transforms the equation into:
まず、分配法則を適用する。これは$1-R_\text{SM}(T_\text{service})$を和の中の各項と乗算する。そうすることで次の式を得る。
$$
\sum_\text{i=0}^{n-1}\left[R_\text{SM}(t-(i+1)T_\text{service})-R_\text{SM}(T_\text{service})
R_\text{SM}(t-(i+1)T_\text{service})\right]
$$
Next, we apply the product rule for reliability functions, which is $
R_\text{SM}(a)R_\text{SM}(b)=R_\text{SM}(a+b) $. It's important to remember that this rule
applies only to terms in the form of $ R_\text{SM} $. We can apply this rule to the
second term in the expanded equation:
次に、信頼性関数の積則を適用する。$R_\text{SM}(a)R_\text{SM}(b)=R_\text{SM}(a+b)$である。$R_\text{SM}$の形の項だけに適用されるので、この規則を展開された方程式の第2項に適用できる。
$$
\sum_\text{i=0}^{n-1}\left[R_\text{SM}(t-(i+1)T_\text{service})-R_\text{SM}(T_\text{service}+t-
(i+1)T_\text{service})\right]
$$
Upon simplifying further, the equation becomes:
さらに単純化すると方程式は次のようになる。
$$
\sum_\text{i=0}^{n-1}\left[R_\text{SM}(t-(i+1)T_\text{service})-R_\text{SM}(t-iT_\text{service})\right]
$$
We can separate this into two distinct summations by splitting the terms within the
summation:
我々は項を分割し2つの異なる和に分けることができる。
$$
\sum_\text{i=0}^{n-1}R_\text{SM}(t-(i+1)T_\text{service})-\sum_\text{i=0}^{n-1}R_\text{SM}(t-iT_\text{service})
$$
Now, to further transform the equation, we change the variable in the first
summation from $i$ to $j=i+1$. This results in adjusting the limits of the
summation as well. The transformed equation becomes:
この方程式をさらに変形するために、最初の和の変数を$i$から$j=i+1$に変更すると変換された方程式は次のようになる。
$$
\sum_\text{j=1}^{n} R_\text{SM}(t - jT_\text{service}) - \sum_\text{i=0}^{n-1} R_\text{SM}(t -
iT_\text{service})
$$
We observe that for each term in the first summation, there is a corresponding term
in the second summation that cancels it out, except for the terms at the boundaries.
What remains are the terms for $j=n$ in the first summation and $i=0$ in the
second summation. Therefore, the simplified result of the equation is:
最初の和の各項について、それを打ち消す2番目の和の対応する項があることがわかる。
残るのは、最初の和の$j=n$の項と、2番目の和の$i=0$の項である。
よって、この式を簡略化すると次のようになる。
$$
R_\text{SM}(t-nT_\text{service})-R_\text{SM}(t)
$$
This represents the remaining terms after all other terms in the summations have
canceled each other out.
これは、和の他の項がすべて相殺された後の残りの項を表している。
ここでこの結果を元の式(13)に代入し、$u\equiv t \bmod T_\text{service}=t-nT_\text{service}$とおけば、 $$ \begin{eqnarray} A_\text{SM}(t)&=&R_\text{SM}(t)+K_\text{SM,MPF}\left[R_\text{SM}(u)-R_\text{SM}(t)\right]\\ &=&(1-K_\text{SM,MPF})R_\text{SM}(t)+K_\text{SM,MPF}R_\text{SM}(u),\ \ \ \text{s.t. }u\equiv t \bmod T_\text{service} \end{eqnarray} $$ これにより論文(13)の最後の式が導出されました。
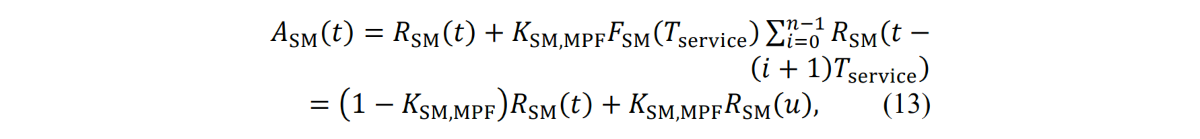

Leave a Comment